|
| |
Virtual Processes as Prior Generative Levels
Potentials from Virtual Particle Exchange
- Where does the Hamiltonian come from? We cannot just invent it!
- We know that the potential energy part of the Hamiltonian really comes
from field-theoretic virtual processes. What are these events?
- Kinetic energy, also, has a mass which is ‘dressed’ by virtual
processes.
- Propose: the Energy Operator is itself ‘generated’ by (further)
previous levels.
Propensities for Virtual Processes
- Propose: 2 linked sets each of three generative levels
- both with (broadly) corresponding processes,
- i.e. still in pattern ‘Principle » Cause » Effect’.
- Virtual processes (in some way) ‘generate’ the terms of the Energy
Operator (the Hamiltonian).

Virtual ‘Principle » Cause » Effect’
- The field-theoretic Lagrangian + Variational Principle starts the
generative sequence.
- Propagating field quanta (virtual quantum field substances),
- e.g. photons, gluons, quarks, leptons, ...
- derived from the Lagrangian by a Variational Principle.
- generate virtual events when interacting.
- Virtual events (of quantum field theory) are point events which
generate the potential energy part of the Hamiltonian operator.
- They do not all actually occur because, for example, they may
generate potentials that are never active in the selected sequence of
actual outcomes.
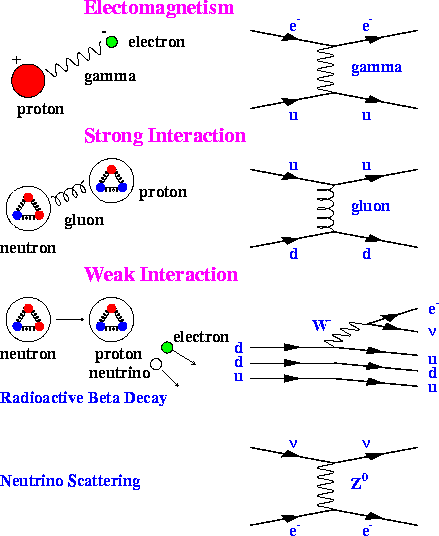
Virtual and Actual Events
|
VIRTUAL EVENTS
|
ACTUAL EVENTS
|
-
Point events (not=point measurements)
-
Interactions
-
Microscopic interactions
-
Continuous
-
Deterministic (apparently)
-
Contribute to alternate futures
-
Have intrinsic group structure (e.g. gauge invariance, renormalisation)
|
-
Visible events in history (e.g. measurement)
-
Selections
-
Macroscopic decoherence
-
Discrete
-
Probabilistic
-
Definitely occur (or not)
-
Have branching tree structure
|
Many possible virtual events contribute to given actual process, such
as electron-electron scattering:
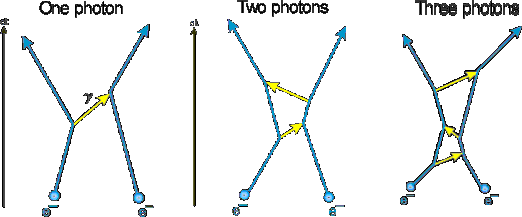
Complications: are all the stages needed?
- Some physicists try to derive probabilities of actual outcomes directly
from field theory, without a Hamiltonian or potential. Is the idea of a
potential only an approximation suitable for some energy scales?
- I would ask: Are there not still some roles for mass, kinetic and potential
energy, & energy conservation?
- I agree that a Hamiltonian (etc) is a ‘composite object’, whose detail
reflects its genesis:
Summary
- More work needed to understand the mathematical substructures at each level,
- But 'virtual processes' do appear to be a level of processes that generates
the Hamiltonians for normal quantum objects.
|
